What Is Z2 Symmetry . generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. please note that below we use the full symmetry group of icosahedral, which includes inversion. So, in this case, the neutral or even state is denoted as +1,. the z_2 symmetry is not an additive symmetry, but multiplicative. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code.
from lessonfullundernotes.z21.web.core.windows.net
the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. the z_2 symmetry is not an additive symmetry, but multiplicative. So, in this case, the neutral or even state is denoted as +1,. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. please note that below we use the full symmetry group of icosahedral, which includes inversion.
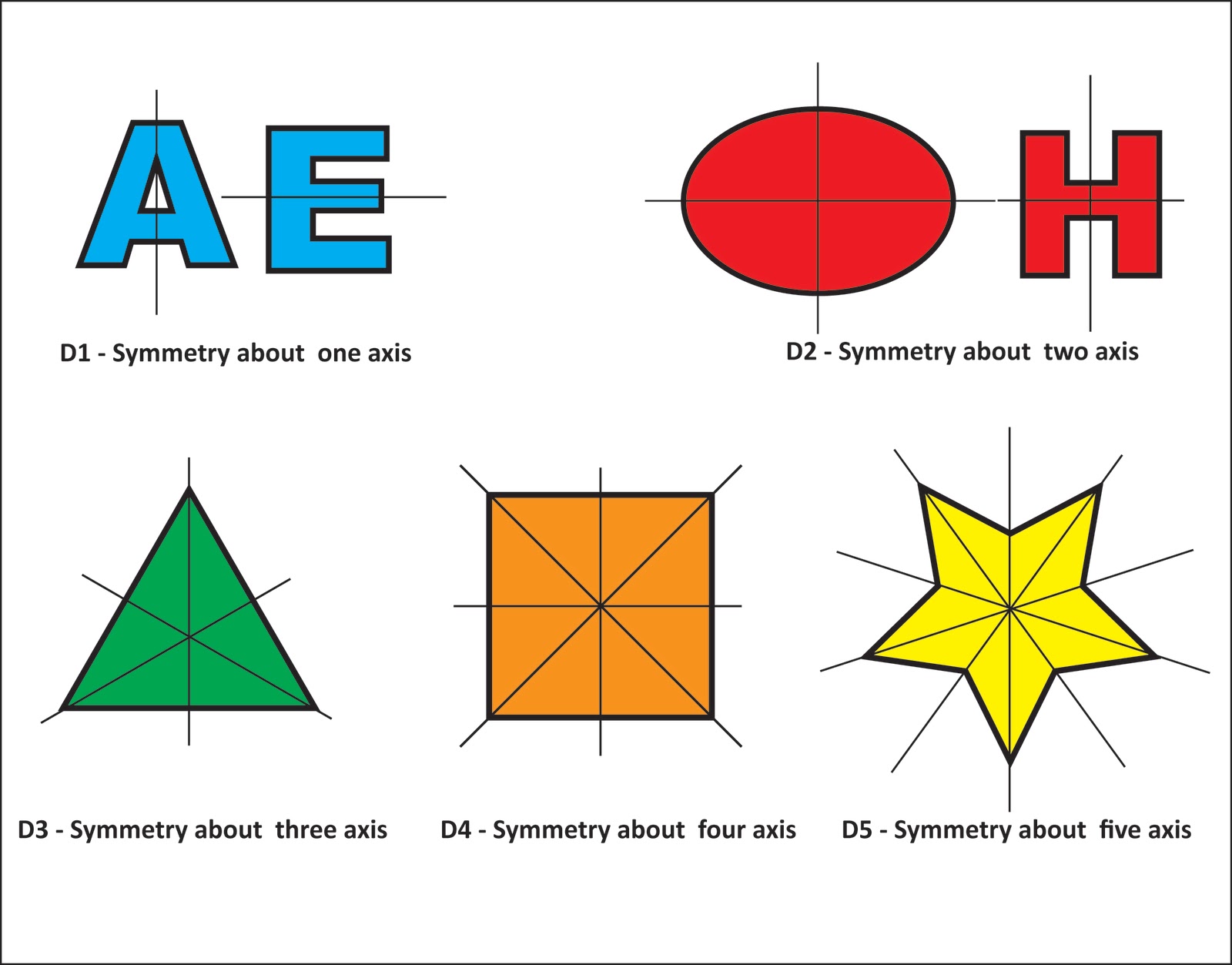
Figures With Lines Of Symmetry
What Is Z2 Symmetry So, in this case, the neutral or even state is denoted as +1,. the z_2 symmetry is not an additive symmetry, but multiplicative. So, in this case, the neutral or even state is denoted as +1,. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. please note that below we use the full symmetry group of icosahedral, which includes inversion. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e.
From www.researchgate.net
Singleline RG fixed point tensor has a Z2 symmetry on the virtual... Download Scientific Diagram What Is Z2 Symmetry the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. So, in this case, the neutral or even state is denoted as +1,. please note that below we use the full symmetry group of icosahedral, which includes. What Is Z2 Symmetry.
From www.semanticscholar.org
Figure 2 from The Calculation of the Hopf/Hopf Mode Interaction Point in Problems with Z2 What Is Z2 Symmetry the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. the z_2 symmetry is not an additive symmetry, but multiplicative. So, in this case, the neutral or even state is denoted as +1,. please note that. What Is Z2 Symmetry.
From www.researchgate.net
Z2 symmetry breaking. a Pump protocol starting in the DW phase, tuning... Download Scientific What Is Z2 Symmetry the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. So, in this case, the neutral or even state is denoted as +1,. please note that below we use the full symmetry group of icosahedral, which includes inversion. the z_2 symmetry is not an additive symmetry, but multiplicative. generally, such a. What Is Z2 Symmetry.
From www.researchgate.net
Power spectrum for a Z2symmetrybroken limit cycle at (δ, W ) = (0.42,... Download Scientific What Is Z2 Symmetry generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. the z_2 symmetry is not an additive symmetry, but multiplicative. please note that below we use the full symmetry group of icosahedral, which includes inversion. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. So,. What Is Z2 Symmetry.
From lessonfullundernotes.z21.web.core.windows.net
Figures With Lines Of Symmetry What Is Z2 Symmetry So, in this case, the neutral or even state is denoted as +1,. the z_2 symmetry is not an additive symmetry, but multiplicative. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. please note that. What Is Z2 Symmetry.
From www.researchgate.net
(PDF) Spontaneous Z2 Symmetry Breaking in the Orbifold Daughter of N=1 Super YangMills Theory What Is Z2 Symmetry please note that below we use the full symmetry group of icosahedral, which includes inversion. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. So, in this case, the neutral or even state is denoted as +1,. the z_2 symmetry is not an additive symmetry, but multiplicative. the local $z_2$. What Is Z2 Symmetry.
From www.researchgate.net
Z2 classification of 1D topological classes with type C symmetry (class... Download Scientific What Is Z2 Symmetry generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. the z_2 symmetry is not an additive symmetry, but multiplicative. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. please note that below we use the full symmetry group of icosahedral, which includes inversion. So,. What Is Z2 Symmetry.
From www.bilibili.com
Z2 TIs with TimeReversal Symmetry 哔哩哔哩 What Is Z2 Symmetry the z_2 symmetry is not an additive symmetry, but multiplicative. So, in this case, the neutral or even state is denoted as +1,. please note that below we use the full symmetry group of icosahedral, which includes inversion. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. generally, such a. What Is Z2 Symmetry.
From www.slideserve.com
PPT Excursions in Modern Mathematics Sixth Edition PowerPoint Presentation ID529142 What Is Z2 Symmetry generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. So, in this case, the neutral or even state is denoted as +1,. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. please note that below we use the full symmetry group of icosahedral, which includes. What Is Z2 Symmetry.
From www.researchgate.net
a) Local Z2 symmetry action on a symmetric TPS (b) changes only tensors... Download Scientific What Is Z2 Symmetry generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. So, in this case, the neutral or even state is denoted as +1,. please note that below we use the full symmetry group of icosahedral, which includes inversion. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric. What Is Z2 Symmetry.
From www.mdpi.com
Symmetry Free FullText Circuit Complexity in Z2 EEFT What Is Z2 Symmetry please note that below we use the full symmetry group of icosahedral, which includes inversion. the z_2 symmetry is not an additive symmetry, but multiplicative. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. So,. What Is Z2 Symmetry.
From www.youtube.com
Group Theory 5c z2 symmetric group YouTube What Is Z2 Symmetry generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. So, in this case, the neutral or even state is denoted as +1,. please note that below we use the full symmetry group of icosahedral, which includes inversion. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric. What Is Z2 Symmetry.
From www.researchgate.net
Dynamics of a Z2 symmetric EdGB gravity in spherical symmetry What Is Z2 Symmetry the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. So, in this case, the neutral or even state is denoted as +1,. please note that below we use the full symmetry group of icosahedral, which includes inversion. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory,. What Is Z2 Symmetry.
From www.researchgate.net
Virtual symmetries of the doublesemion doubleline tensor. It has the... Download Scientific What Is Z2 Symmetry generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. please note that below we use the full symmetry group of icosahedral, which includes inversion. the z_2 symmetry is not an additive symmetry, but multiplicative. So,. What Is Z2 Symmetry.
From www.slideserve.com
PPT Quark number density in the phase with u nbroken Z2 symmetry in twoflavor QCD PowerPoint What Is Z2 Symmetry the z_2 symmetry is not an additive symmetry, but multiplicative. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. please note that below we use the full symmetry group of icosahedral, which includes inversion. So, in this case, the neutral or even state is denoted as +1,. the local $z_2$. What Is Z2 Symmetry.
From zhuanlan.zhihu.com
Time Reversal Symmetry & the Z2 Index 学习笔记 知乎 What Is Z2 Symmetry the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. So, in this case, the neutral or even state is denoted as +1,. the z_2 symmetry is not an additive symmetry, but multiplicative. please note that below we use the full symmetry group of icosahedral, which includes inversion. generally, such a. What Is Z2 Symmetry.
From www.researchgate.net
Download PDF Adiabatic preparation of maximum entanglement in hybrid quantum systems with the What Is Z2 Symmetry the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. the z_2 symmetry is not an additive symmetry, but multiplicative. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. please note that below we use the full symmetry group of icosahedral, which includes inversion. So,. What Is Z2 Symmetry.
From www.researchgate.net
The phase diagram of the model (51) with Z2 × Z2 symmetry. The black... Download Scientific What Is Z2 Symmetry the z_2 symmetry is not an additive symmetry, but multiplicative. So, in this case, the neutral or even state is denoted as +1,. generally, such a statement implies that there is a $\mathbb{z}_2$ symmetry of the theory, i.e. the local $z_2$ gauge symmetry operator is essentially the plaquette term of the toric code. please note that. What Is Z2 Symmetry.